Bridge Height
All the time I am asked “How tall should I make my bridge?” This article will attempt to answer this question by illustrating a principle in model bridge building. There is no cut and dry answer, as you should evaluate your bridge specifications and guidelines and conduct experiments to reach the best answer for you.
h3>How Changing Height Affects a Bridge
Control Bridge
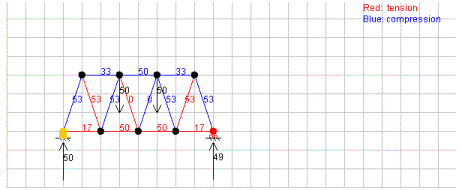
This is our control bridge. The bridge is 8 inches long and 3 inches tall. I have added two load points with a total load of 100. This means the numbers you see act as percentages. For instance, the very middle of the top chord of the bridge is supporting 50% of the total load.
Now I will show you another bridge, with the same design. The only difference is that this bridge is 4 inches tall, one inch taller than before.
1 inch taller
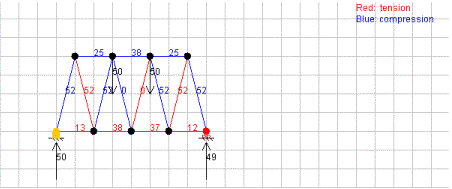
You can see in this second bridge that the middle section of the top chord is only holding 38% of the total load. All I did is increase the height of the bridge by one inch.
What does this mean to you?
As you saw in the example bridges, by increasing the height of the bridge you decrease the load on the top (and bottom) chord. A decrease in load means you can make it smaller. Smaller means lighter in this case. However, there is a catch.
Look back at the two examples. By increasing the height, the load decreased on the top and bottom chords but remained the same on the middle “truss members”. Why is this a drawback? By increasing the height, the middle members have to become longer. That inherently adds weight. However, bridge builders have another problem when pieces become longer. The amount a piece of wood can support in compression before buckling decreases with length. So that means the middle members will have to be made stronger the taller the bridge is. Adding strength usually means adding weight.
-
- 3 inches taller
-
- Two inches taller
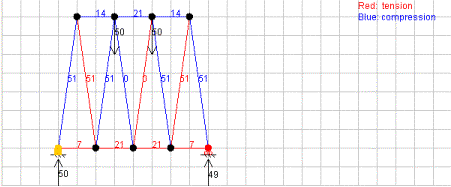
So we have a draw
By increasing the height of a bridge, you can make the top and bottom chords lighter. But at the same time, the middle truss members have to be made heavier. The goal is to find a balance. I believe that there is an optimal height for a model bridge in every situation. The trick is to find that height, by balancing out these two factors. I was once told that the optimal height for an arch bridge is 1/6th the length of the span. That means if your bridge is 6 inches long, it should be 1 inch tall. My experience has confirmed that this is a very good starting place for an arch bridge. And I believe the same ratio of height to length can be applied to regular truss bridges.
The Optimal Height
Interestingly, the Golden Gate Bridge uses the 1/6th ratio almost exactly. Perhaps you can do some studying on other real bridges and see if they follow this idea also.
Data taken from the Bridge Designer.
what would be a good formula or rule of thumb to chose the number of trusses for building a model bridge?
The sketches are backwards, the information in them needs to be swapped. (just incase anyone gets confused at first glance…)
The sketches do not need to be switched. They are exactly as I want them.
the post on Bridge Height tackles the load distribution in rising the bridge. You talk about the bridge in terms of total length versus total height. But what about how many diagonals are necessary for a given lenght? Or how low can the angle of these diagonals be? (the less diagonals, the less material necessary)
Does the 6:1 ration apply to bridges that are elevated? I’m doing the SciOly elevated bridge event for division C.
Thanks for the post. I have a backyard project that needs a 25 foot bridge. (mostly decorative, but would be embarrasing to have it collapse) The math is what I was lacking.
Is an arch bridge more efficient than a truss bridge?
Also, in a situation where the ratio is at most 2:1, what kind of bridge should be built?
I need to make a strong, light bridge for my class. Is the K-Beam the best option, and if so, what is the best way to build this? I REALLY need the info for my eighth grade elective! The bridge must be 14-16in. long, 4in. wide, and 4-6 in. long.